Formula Student aerodynamics

In this post I’m going to evaluate the aerodynamics a Formula Student car. The vehicle geometry was kindly provided by one of the teams, participating in Formula Student Germany, for a free online workshop on the Application of CFD in Formula Student and Formula SAE hosted by SimScale.

Although the geometry is based on an real Formula Student car, the configuration used for the simulation is slightly different from that of the actual car. For instance, the position of the rear wing appears to be higher in the simulation. So, the results shown here are not representative of the actual performance of the car.

Simulation setup

The CAD geometry is freely available at SimScale, as well as the set of instructions that will get you through all the steps of setting up a case: from the mesh generation to the post-processing of results. The final mesh has close to 8 million cells, with a porous zone for the radiator and a MRF (multiple reference frame) zone for the wheels.

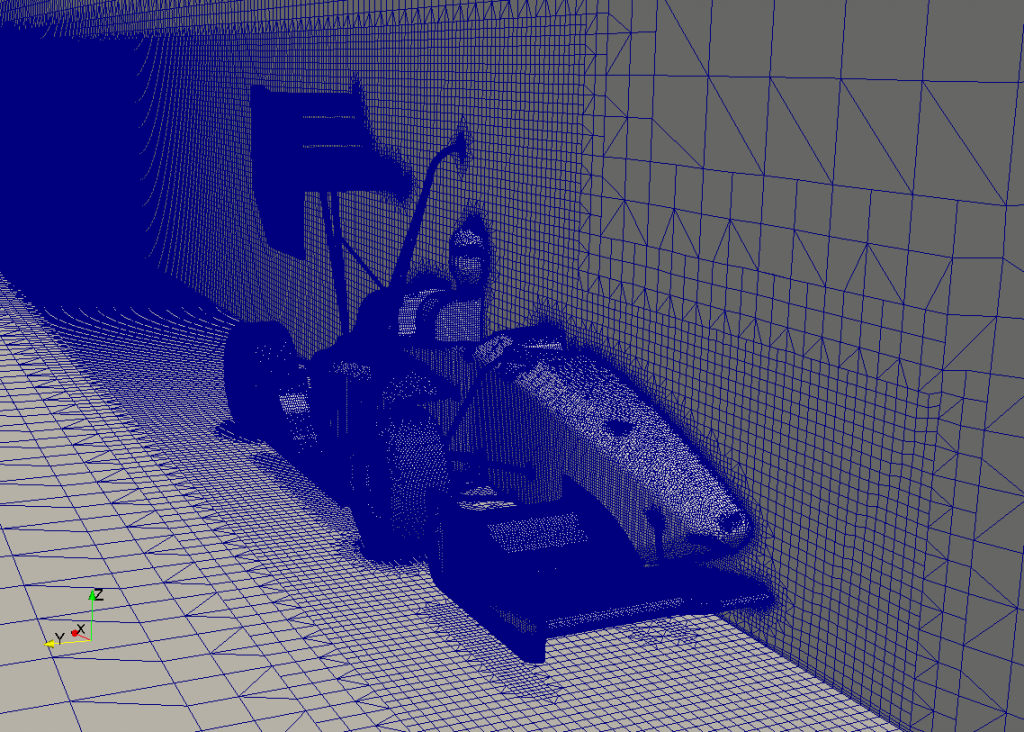
The CFD simulation is RANS based with the well-known k-omega SST turbulence model. Inlet velocity is set at 20 m/s (72 km/h), which is quite reasonable considering the kind of competitions this Formula Student vehicles are involved in.

Results

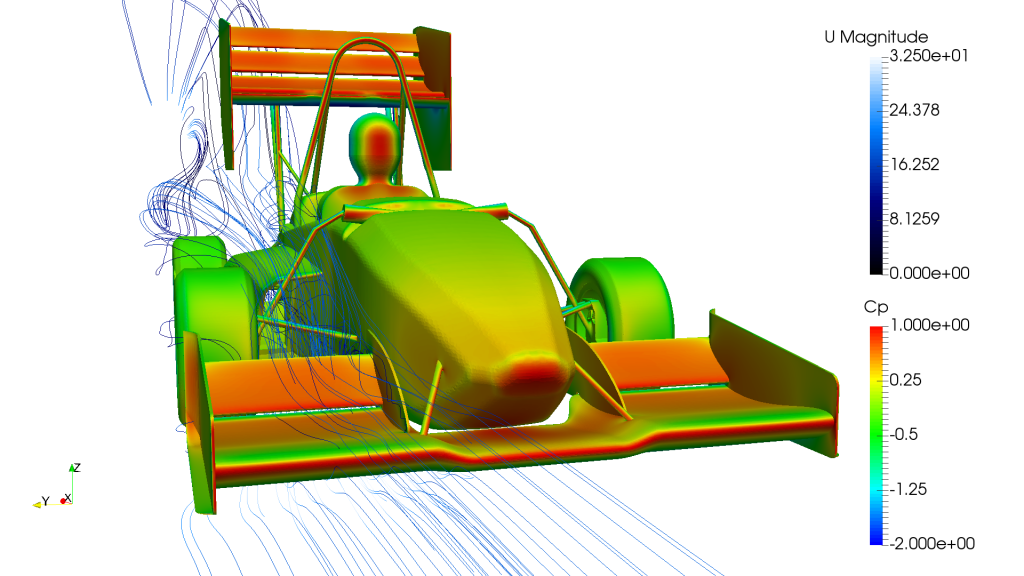
Pressure contours on the surface of the car are the most common post-processing visualizations found on CFD. When combined with the jet colormap we are all used to we can identify high pressure areas (red) and low pressure areas (blue).

Drag

In order to find out whether those high and low pressures contribute to increasing the drag, we have to consider the orientation of the surface. For that, it’s useful to decompose the pressure on its three Cartesian components and plot only the pressure along the x-axis. In the pictures below we can see that the front wing flap, the driver, and the rear wing elements are the main contributors to pressure drag.

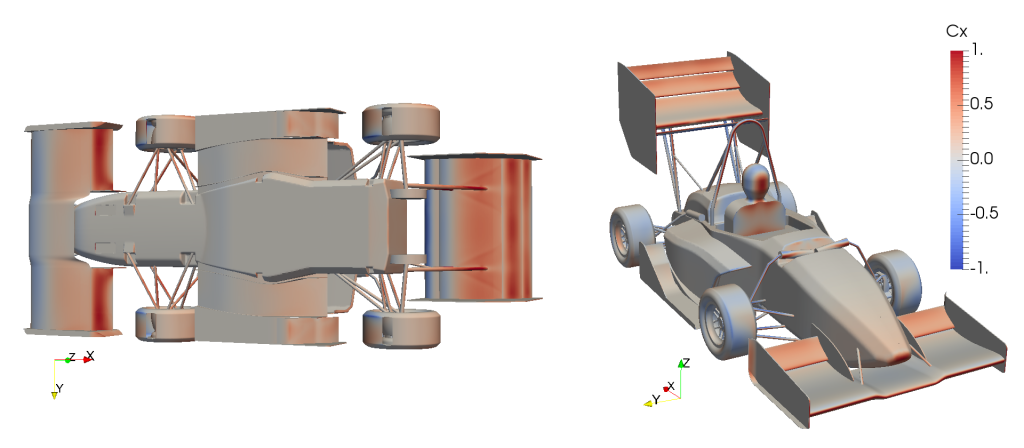
A good deal of the wheels is of a blue-ish color meaning that the front wing and the diffuser are deflecting the incoming air creating areas of low pressure ahead of the wheels where we would normally get stagnation (See this other project for a reference of a Formula Student car without aerodynamic elements).

Another interesting post-processing technique is the accumulated drag making use of the bins available since OpenFOAM 2.2.0. We should be careful with this though as, technically speaking, pressure drag makes sense as drag only after it’s been integrated over the surface of a closed body (McLean, 2012).

It is readily apparent the wings are responsible for most of the drag. That is, downforce comes at a cost.

Downforce

The same analysis can be applied to investigate downforce (negative lift). In the picture below, the blue colour represents a contribution to downforce, while red indicates lift.

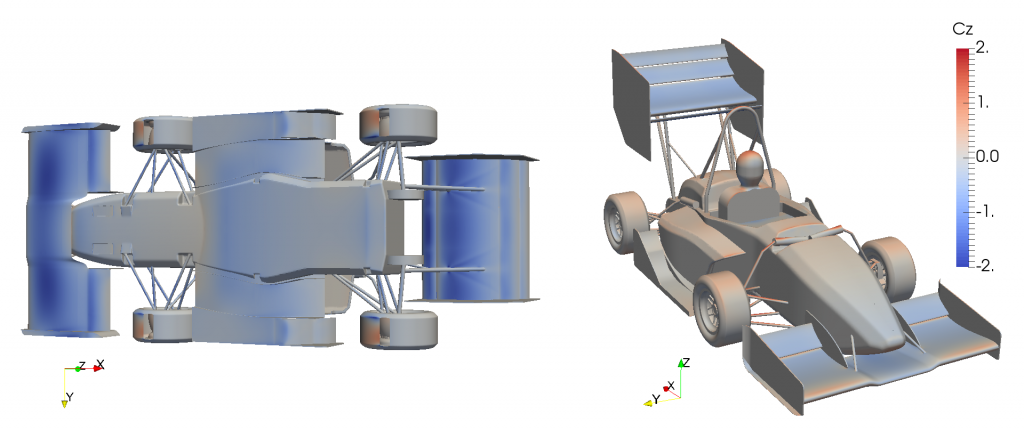
It is no surprise that a great deal of downforce comes from both wings. On the contrary, the diffuser is not as effective in this case as one would imagine.

In the case of downforce I do not use the accumulated value, but just the bins themselves. In this particular case, if we group the data into 4 bins we get them nicely distributed so that: the first bin lies on the front wing area, the third bin is just around the diffuser, and the fourth bin accounts for the rear wing.

The front wing area generates the most downforce, followed by the rear wing. As already anticipated from the images, the diffuser area is generating much less downforce. The distribution of downforce for each bin is as follows:

44.3 %

1.0 %

13.7 %

42.0 %

How much of that downforce is transmitted to each wheel could be easily calculated, knowing the relative position of each wheel along the x-axis, by applying basic beam analysis techniques.

Total pressure

Total pressure can be considered an indicator of the energy of the flow and is useful to identify fluid structures. Some of those structures can be seen in the following animation.

In the following video, Torbjörn Larsson —former Head of CFD at Sauber, BMW, and Ferrari— explains some other post processing techniques:

References

McBeath, S. (2015). Competition car aerodynamics: a practical handbook (New 3rd ed). Poundbury, Dorchester: Veloce Publishing.

McLean, J. D. (2012). Understanding aerodynamics: arguing from the real physics (1st ed.). Chichester: Wiley.